Right Triangular Prism Volume Calculator
| Result: |
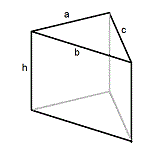
Volume of a Right triangular prism = Area of triangular face * height
Choose the shape you want to calculate the volume of:
Right Triangular Prism Volume Calculator
Introduction
Understanding the volume of three-dimensional shapes is crucial in various fields, including engineering, architecture, and education. Our Prism Volume Calculator simplifies calculating the volume of right triangular prisms, making it accessible for everyone from students to professionals.
What Is a Right Triangular Prism?
A right triangular prism is a three-dimensional solid having two congruent right triangles as its bases and rectangles as its sides. It's a type of polyhedron that is widely used in real-life applications, such as in architectural designs and various engineering projects.
How Does It Work?
Our calculator uses the formula for the volume of a right triangular prism: \( V = A_b \times h \), where \(A_b\) is the area of the base triangle and \(h\) is the height (length) of the prism. The area of the base triangle is calculated using the formula \( A = \frac{1}{2} \times base \times height \) of the triangle.
Formula in Use
The volume of a right triangular prism can be computed as:
\[ V = \frac{1}{2} \times a \times b \times h \]
Here, \(a\) and \(b\) are the sides of the right triangle forming the base, and \(h\) is the height of the prism.
Uses and Applications
Right triangular prisms are prevalent in construction and design. Architects and engineers use the volume of these prisms to calculate materials needed for building structures, while educators use them to teach geometric concepts in classrooms.
Frequently Asked Questions on Prism Volume Calculator
- How do you find the volume of a right triangular prism?
The volume is calculated by multiplying the area of the base right triangle by the prism's height, using the formula: \( \text{Volume} = \frac{1}{2} \times \text{base} \times \text{height} \times \text{prism height} \). - What is the volume of a right triangular prism calculator?
It is an online tool that computes the volume of a right triangular prism given the dimensions of the base triangle and the prism's height. - What is the formula for the volume of a right triangular triangle?
The formula for a right triangular prism is: \( \text{Volume} = \frac{1}{2} \times \text{base} \times \text{height} \times \text{prism height} \), where base and height are the dimensions of the triangle. - What is the formula for the volume of a right prism?
The volume of a right prism is calculated as: \( \text{Volume} = \text{base area} \times \text{height} \), where the base area is determined by the shape of the prism's base. - What is a right triangular prism?
A right triangular prism is a three-dimensional solid with two congruent right triangles as its bases and rectangular faces as its sides. - What is the area of a right triangular prism?
The surface area is the sum of the areas of the two triangular bases and the areas of the three rectangular faces. - What is the volume of the right prism pyramid?
This may be a confusion between shapes. A prism's volume is base area times height, whereas a pyramid uses \( \frac{1}{3} \) base area times height. - What is the volume of a right prism square?
For a square-based right prism, the volume is the side length squared times the height of the prism. - What is the volume of triangle Class 8?
Triangles do not have volume as they are two-dimensional shapes. This might refer to the volume of triangular prisms or pyramids. - What is the formula for the volume of pyramids?
The volume formula for a pyramid is \( \text{Volume} = \frac{1}{3} \times \text{base area} \times \text{height} \). - What is a triangular prism with 3 sides?
A triangular prism has two triangular bases connected by three rectangular faces. - What are volume formulas?
Volume formulas are used to calculate the space occupied by a three-dimensional object, based on its shape and dimensions. - What is base area?
Base area refers to the area of the shape that forms the bottom or top surface of a three-dimensional object. - How to find the volume?
To find the volume, apply the shape-specific formula, often involving multiplying the base area by the height. - Is a cone a pyramid or prism?
A cone is neither a pyramid nor a prism. It is a unique three-dimensional shape with a circular base tapering to a point.