Cuboid Volume Calculator
| Result: |
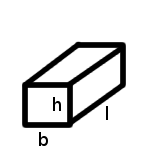
Volume of a Cuboid formula = l * b * h
Choose the shape you want to calculate the volume of:
Cuboid Volume Calculator
Understanding how to calculate the volume of a cuboid is a fundamental skill useful in various real-life scenarios. This calculator is designed to make these calculations straightforward for everyone.
What is a Cuboid?
A cuboid is a three-dimensional geometric figure with six rectangular faces. Examples include boxes, aquariums, or rooms.
Volume of a Cuboid Formula
The volume of a cuboid is calculated by multiplying its length (\(l\)), width (\(b\)), and height (\(h\)). The formula is:
\[ \text{Volume} = l \times b \times h \]
Frequently Asked Questions
How do you calculate the volume of a cuboid?
Multiply the length, width, and height of the cuboid. The formula is \(\text{Volume} = l \times b \times h\).
What is the volume of a cuboid 80cm long, 60cm wide, and 10cm deep?
The volume is \[80 \times 60 \times 10 = 48000\] cubic centimeters.
What is the area formula of a cuboid?
The surface area of a cuboid is calculated as: \[2(lb + bh + hl)\], where \(l\) is the length, \(b\) is the breadth, and \(h\) is the height.
What is the volume of a cuboid example?
For a cuboid with dimensions 6cm, 8cm, and 10cm, the volume is: \[6 \times 8 \times 10 = 480\] cubic centimeters.
What is the formula of volume?
For a cuboid, the volume formula is \[V = l \times b \times h\]. This formula varies based on the shape being considered.
How to find the volume?
Identify the shape of the object, use the corresponding volume formula, and plug in the dimensions into the formula.
What is the volume of a cuboid 6cm by 8cm by 10cm?
The volume is \[6 \times 8 \times 10 = 480\] cubic centimeters.
What is the volume of a cuboid 10cm by 3cm by 2cm?
The volume is \[10 \times 3 \times 2 = 60\] cubic centimeters.
What is the volume of a cuboid 4cm by 2cm by 3cm?
The volume is \[4 \times 2 \times 3 = 24\] cubic centimeters.
What is the formula for calculating volume?
The general formula for volume depends on the shape of the object. For cuboids, it is \[V = l \times b \times h\].
What is the volume of a cuboid in year 6?
In year 6 education, the focus is on understanding the basic concept of volume as \[V = l \times b \times h\], using simple numerical examples.
What is the volume of a cuboid with an example and solution?
For a cuboid with dimensions of length = 5cm, width = 4cm, and height = 3cm, the volume is \[5 \times 4 \times 3 = 60\] cubic centimeters.